Editor Aparigraha Jain
Editor Carla Geerdes
When we build a Moebius Strip, we made the plane (strip of paper) go through 3D space by twisting it.
- The Moebius Strip is a curved plane in 3D.
Now lets press the moebius strip flat on the table.
In left picture we see a hole like a triangle in the middle.
To avoid this we have to shorten the length of the strip.
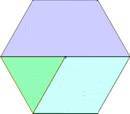
Without the hole in the midle we get this view:
Discover:
Its a hexagon - containing the sequence: 1-2-3
- 1 triangle (green)
- 2 triangles (light green)
- 3 triangles (violet)
A hexagon designed like this
represents a cube (3D space), seen from a specific standpoint.
For the Creatives
Here is a design for reconstruction:
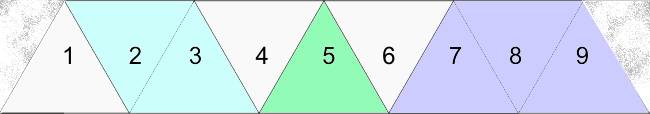
save the picture (it comes full size), print it, cut out the strip and tape it (glue #1 on #9)
Fold the strip along the triangle lines, where the colours change.
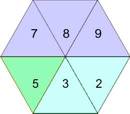
Tessellation
from above
moebius strip design
Try yourself
Hexagons have 6 equilateral triangles inside (each side has the same length).
The angel between 2 sides is 60°.
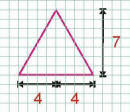
If you use squared paper for your own ideas
you can define the 3 edges of an equilateral triangle
by counting the squares.
2 examples for reconstruction
The background for these designs is a "knot" structure.
Knots too have their specific systems building networks by connecting lines (1D) to planes (2D), including space(3D) into their construction process.
A lot of knowledge about dimensions, their visual perception and meaning is hidden in these old techniques.